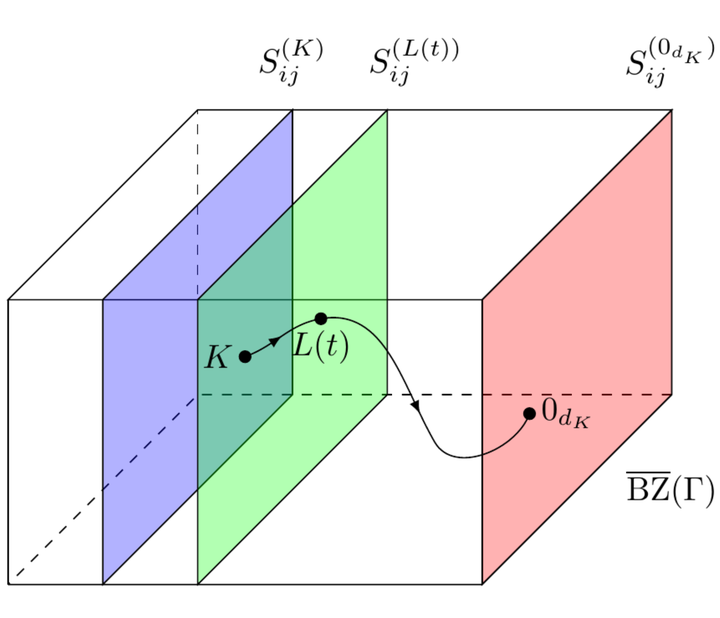
Abstract
We establish a connection between the electromagnetic Hall response and band topological invariants in hyperbolic Chern insulators by deriving a hyperbolic analog of the Thouless-Kohmoto-Nightingale-den Nijs (TKNN) formula. By generalizing the Kubo formula to hyperbolic lattices, we show that the Hall conductivity is quantized to $−e^2C_{ij}/h$, where $C_{ij}$ is the first Chern number. Through a flux-threading argument, we provide an interpretation of the Chern number as a topological invariant in hyperbolic band theory. We demonstrate that, although it receives contributions from both Abelian and non-Abelian Bloch states, the Chern number can be calculated solely from Abelian states, resulting in a tremendous simplification of the topological band theory. Finally, we verify our results numerically by computing various Chern numbers in the hyperbolic Haldane model.